Easy Way to Remember Real Estate Math

Real estate math is an essential part of the real estate exam and an important concept to understand to have a successful real estate career. Becoming an expert at math and being able to do real estate math problems can help you stand out in your market and become a better real estate agent and can make it much easier to pass the real estate exam.

How Much Math Is on the Real Estate Exam?

No matter what state you are wanting to get a real estate license in, you can expect to see math questions on the exam. While the number of math questions on the exam varies from state-to-state, the total number of math-related questions is somewhere between 10-15%.

How Is Math Used in Real Estate?

While you may not need to use math every day as a real estate agent, you should be prepared when problems arise that require a thorough understanding of real estate math concepts. Examples of math concepts that real estate agents must know are as follows:.

• Measurement Conversions: Measurements including area measurements, linear measurements, and volume measurements
• Fractions, Decimals, & Percentages: These include understanding The T-Bar Method or how to solve percentage problems
• Real Estate Math Formulas: Math formulas help you solve problems you'll encounter frequently as an agent. These include the Gross Rent Multiplier (GRM) Formula, the Commission Formula, Simple Interest Formula, Loan to Value Ratio (LTV), and more.

Is Real Estate Math Difficult?

Real estate math is NOT difficult. Many students dread the idea of learning math and having to use math in their careers, however, real estate math is not challenging and there are only a few concepts that you need to master. The more practice and time spent on understanding the math problems and concepts that you may see, the better you will do on the exam and throughout your career.

Real Estate Math Definitions

Basic Arithmetic Skills Vocabulary

Term	Definition
Baseline	A measured line through a survey area from which triangulations are made
Benchmark	A surveyor's mark made on a stationary object of previously determined position and elevation and used as a reference point in tidal observations and surveys.
Board Foot	A unit of cubic measure for lumber, equal to one foot square by one inch thick.
Decimal	Pertaining to tenths or to the number 10. The symbol that creates a decimal is called the decimal point. In the number 125.67, the period between the 5 and the 6 is called the decimal point.
Denominator	The expression written below the line in a common fraction that indicates the number of parts into which one whole is divided. For example, in the fraction 3/5, 5 is the denominator. In the number 125 3/5, 5 is the denominator. The term refers only to the bottom number in the fraction, not to the rest of the number.
Equivalent Fraction	Fractions which have the same value, even though they may look different. Example ½ and 2/4 are equivalent because they are both half.
Fraction	An expression that indicates the quotient of two quantities, such as 1/3 A disconnected piece; a fragment.
Front Foot	A method of describing or pricing commercial real estate by the number of feet of road frontage the parcel has. The drawback is that there is no widely recognized standard for depth, so a property selling for $1,500 per front foot might be half the depth of one selling for $2,400 a front foot, but no one can tell just from the price.
Governmental Survey System/Rectangular Survey System:	A system of dividing land in the United States into 24-square mile quadrangles from the north-south line and the east-west line.
Greatest Common Factor:	The largest whole number that divides evenly into each of the numbers. For example, the greatest common factor of 4, 8, 12 and 16 is 4, because 4 is the largest number that will divide evenly into each of the numbers. 4÷4=1, 8÷4=2, 12÷4=3, 16÷4=4.
Latitude	The angular distance north or south of the earth's equator, measured in degrees along a meridian, as on a map or globe.
Lineal Foot	The same as a foot. If something is 12 lineal feet long, it is 12 feet long.
Longitude	Angular distance on the earth's surface, measured east or west from the prime meridian at Greenwich, England, to the meridian passing through a position, expressed in degrees (or hours), minutes, and seconds.
Lowest Common Denominator	In mathematics, the lowest common denominator or least common denominator (abbreviated LCD) is the least common multiple of the denominators of a set of fractions. It simplifies adding, subtracting, and comparing fractions.
Meridian	An imaginary great circle on the earth's surface passing through the North and South geographic poles. All points on the same meridian have the same longitude.
Numerator	The top number in a fraction. Shows how many parts are being counted. - Math Is Fun Rod Pierce.
Pi	A transcendental number, approximately 3.14159, represented by the symbol [image], that expresses the ratio of the circumference to the diameter of a circle and appears as a constant in many mathematical expressions
Point of Beginning	The point of beginning is a surveyor's mark at the beginning location for the wide-scale surveying of land.
Product	The answer or result when two or more numbers are multiplied. For example, in 10 × 5 = 50, the product is 50.
Range	A north-south strip of townships, each six miles square, numbered east and west from a specified meridian in a U.S. public land survey.
Rounding	In math, rounding refers to reducing a number (usually the answer to the math problem) to a number shorter than the exact answer the calculation has produced. Very simply, it means using fewer digits in the number while still maintaining a very similar result. To round a number, you first decide which is the last digit you want to use; the more accurate a measurement is necessary, the more digits.
Running Foot	A measurement of the length of a piece of wood, without regard to its thickness or width.
Square Foot	A unit of measurement of an area. A square foot is a surface 12 inches on each side.
Township	A public land surveying unit of 36 sections or 36 square miles.

View more basic real estate math definitions inside our Principles in Real Estate course.

Measurement Conversions

Being able to understand measurements will help you establish a solid foundation for being an expert throughout your real estate career. Below is a list of the measurements and conversions you will need to master.

Linear Measurement Conversions

• 12 inches = 1 foot
• 3 feet = 1 yard
• 1 mile = 5,280 linear feet
• 1 rod = 16 ½ linear feet
• 1 chain = 4 rods
• 4 rods = 100 links
• 1 link = 7.92 inches
• 1 mile = 320 rods
• 1 mill = 0.10 of 1 cent
• 1 hectare = 2.471 acres
• 1 township = 36 sections
• 1 section = 1 square mile
• 1 acre = 43,560 square feet
• 1 acre = 10 square chains
• 360 degrees = full circle
• 90 degrees = ¼ circle
• 1 degree = 60 minutes
• 1 minute = 60 seconds

Area Measurements

Area measurements are given in a variety of different units. The following formulas will refresh your knowledge of these units.

• 144 inches = 1 square foot
  • Number of square inches ÷ 144 = number of square feet
  • Number of square feet × 144 = number of square inches
• 1,296 square inches = 1 square yard
  • Number of square inches ÷ 1,296 = number of square yards
  • Number of square yards × 1,296 = number of square inches
• 9 square feet = 1 square yard
  • Number of square feet ÷ 9 = number of square yards
  • Number of square yards × 9 = number of square feet
• 43,560 square feet = 1 acre
  • Number of square feet ÷ 43,560 = Number of acres
  • Number of acres × 43,560 = Number of square feet
• 640 acres = 1 section = 1 square mile
  • Number of acres ÷ 640 = Number of sections (also Number of square miles)
  • Number of sections (or square miles × 640 = Number of acres

Volume Measurements

As with other means of measuring things, the volume of a space or object can be expressed in a number of different ways.

• 1,728 cubic inches = 1 cubic foot
  • Number of cubic inches ÷ 1,728 = # of cubic feet
  • Number of cubic feet × 1,728 = # of cubic inches

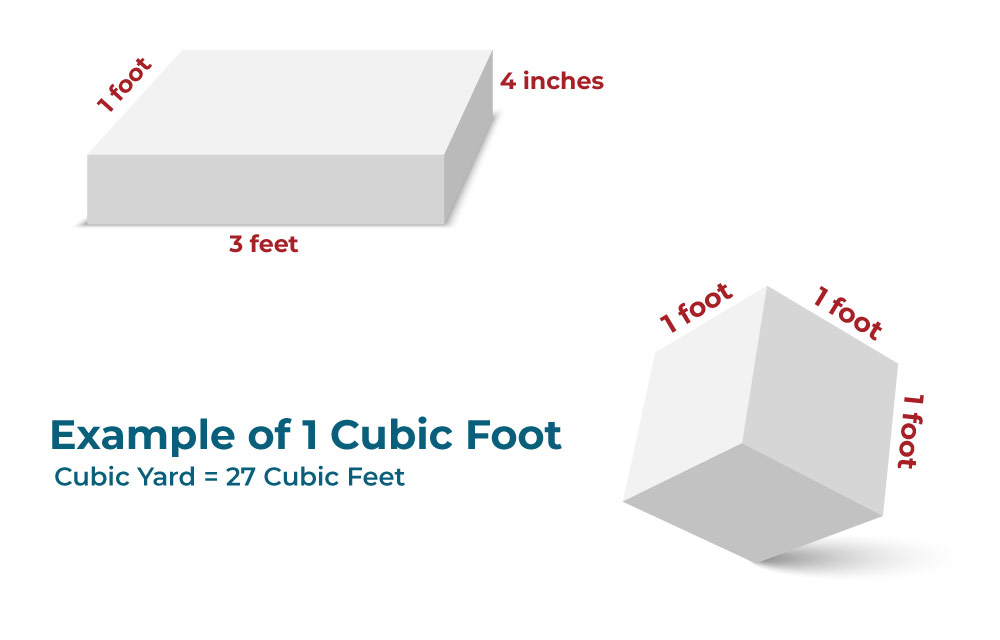
Cubic Foot Diagram

Example Measurement Problem

Your client needs to rent climate-controlled, insured and bonded warehouse space for 6 months to store 500 pallets of construction tools from the largest toolmaker in China, each full pallet being 4 feet by 5 feet by 8 feet tall, and all shrink-wrapped in industrial-grade plastic. The only space that s available in town leases for 22.5 cents/cubic foot/month. What is the cost of the space?

• 4 × 5 × 8 = 160 cubic feet
• 500 × 160 = 80,000 cubic feet
• 80,000 × .225 = $18,000/month
• Answer $18,000 × 6 = $90,000 total cost for six months

Fractions, Decimals, and Percentages

Fractions

A fraction is a part of something. Fractions tell us how many parts the whole is divided into, as well as how many of those parts we are working with. For example, in the fraction ¼, the bottom number called the denominator, tells us the item has been divided into 4 parts; the top number, called the numerator, tells us we are working with 1 of those 4 parts.

Decimals

Fractions are also expressed as decimals. The fraction ¼ can be expressed as .25, the fraction ½ is also expressed as .5, ¾ as .75, and so on. How do you convert a fraction to a decimal fraction? Simply by dividing the top number (numerator) by the bottom number (denominator).

To convert ¾ to the equivalent decimal fraction: 3 ÷ 4 = .75
In the case of ¼, 1÷4 = .25

As a real estate agent or REALTOR® and on the license exam, you will be using a calculator rather than a pencil and paper, so you will almost always find it is easier to convert fractions to decimals before doing the calculations. Calculators are based on decimal points rather than fractions. You can enter 1.25 on the calculator; you cannot enter 1 ¼.

Percentages

A percentage is an expression meaning per hundred or per hundred parts. Therefore, if you say "3%", you are saying that the item being measured has been divided into 100 parts and that the portion you are describing is made up of three of those 100 parts. The placement of the decimal point in the number is important:

• .10 means 1/10 as well as 10 parts per hundred as well as 10%
• .01 means 1/100, as well as 1 part per hundred as well as 1%
• .001 means 1/1000, as well as 1 part per thousand as well as .1%
• .0001 means 1/10,000 as well as 1 part per ten thousand as well as .01%

Solving Percentage Problems

There are three formulas that are important for solving all percentage problems.

1. PART = TOTAL × RATE
2. TOTAL = PART ÷ RATE
3. RATE = PART ÷ TOTAL

Another way to remember these formulas is to think:

• If PART is unknown Multiply.
• If PART is known Divide.
• When you divide, always enter PART into the calculator first.

T-Bar Method

Many real estate students do not feel comfortable with the 3 formulas used to solve percentage problems, so another way to approach this is visualize a 'T', The 'T" will represent the relationship between PART, TOTAL, and RATE. This method is known as the T-Bar Method.

Using the T-Bar method, insert the known figures in the correct places inside the circle. Multiply if the line between the figures is vertical to get the unknown, and divide if the line between the figures is horizontal to get the unknown. If dividing, always input PART first into the calculator.

Real Estate Math T-Bar Method

Real Estate Math Formulas

Math formulas are an essential component to pass the exam and becoming a successful real estate broker or sales agent. Remember, practice makes perfect, so the more time you spend memorizing these formulas, the better off you will be.

Loan to Value (LTV) Ratio

LTV Ratio = APV ÷ MA

APV = Appraised Property Value
MA = Mortgage Amount

Simple Interest Formula

A = P(1 + rt)

A = Total Accrued Amount (principal + interest)
P = Principal Amount
I = Interest Amount
r = Rate of Interest per year in decimal; r = R/100
R = Rate of Interest per year as a percent; R = r * 100
t = Time Period involved in months or years

Gross Rent Multiplier

Gross Rent Multiplier = Property Price ÷ Gross Annual Rental Income
Annual Gross Rental Income = Monthly Rental Income × 12

Gross Rent Multiplier Calculator

Property Tax Formulas

Property Tax Rate = Assessed Value × Mill Rate
Assessed Value = Assessment Market × Market Value
1 mill = 1/1,000th of a dollar or $1 in property tax

Discount Points Formulas

Discount Points = Prepaid Interest
Break-Even Point = Points Cost ÷ Monthly Payment Savings

"The Mortgage Rule of Thumb" (28/36 Rule) Formula

Housing Costs to Qualify for Most Loans = Gross Monthly or Annual Income × .28

Proration

Proration is the name we give to making a fair division of the costs and benefits of a financial transaction. In the context of real estate, we are dealing with larger numbers, and dividing such things as real estate taxes, homeowners' association fees, rents paid by tenants, and so on, but the concept remains the same. The question is, who pays for what, and the proration process helps make that determination.

At closing, various items are prorated and some fees are often shared among the buyer, seller, and brokers. In other words, the total amount must be prorated or allocated according to a proportionate distribution, just like those cookies mentioned above. Typical expense items to be prorated include property taxes, monthly interest due when loans are assumed, rent, and homeowner fees.

Prorating Interest on Loans

Interest is almost always paid in arrears (paid at the end of the period). In other words, when you make your mortgage payment on the first of the month, you are paying the interest portion for the previous month.

Interest on a new loan is calculated by multiplying the principal balance time the interest rate, then dividing by 365 days.

Prorating Interest on Loans Example

The Buyer obtains a new loan in the amount of $150,000.00 at 8% interest.

• Multiply the principal amount times the interest rate to get the annual interest, $150,000.00 times .08 = $12,000.00.
• Now determine the daily or per diem rate by dividing the annual interest by 365, $12,000.00 divided by 365 = $32.876712 per day (per diem).
• The closing is to take place on July 15. July is the period of time being used, and there are 31 days in the period. Therefore, the Buyer will own the property for 17 days in July.

Now It's Time to Start Practicing

In this post, we covered a variety of real estate math topics, math formulas, and basic arithmetic skills that will need to know to pass the real estate exam and have a successful career.

You can also find additional practice questions and problems with our Real Estate Exam Prep Courses, our Principles in Real Estate course, and flashcards.

Principles in Real Estate - Unit 8: Real Estate Math Review Summary Video

Download Math Practice Worksheet

Written and Published by: VanEd

jacksontreadevent77.blogspot.com

Source: https://www.vaned.com/blog/real-estate-math/

Share this post